Standard: 7.C.3: Measurement and Estimation
~ MS/Jr. High
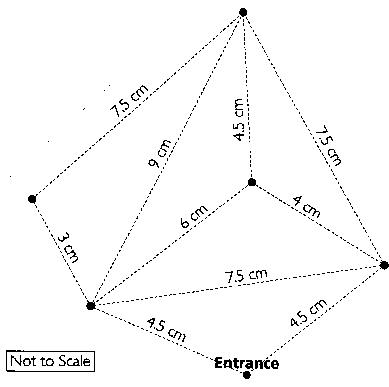
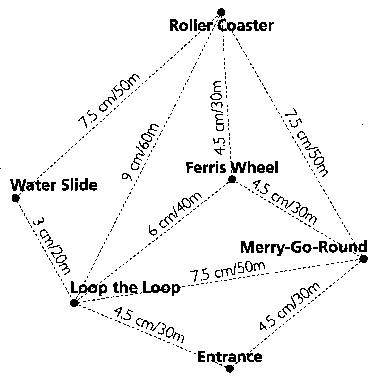
Title: Amusement Park Map
Problem: On the map 3 centimeters stand for 20 meters.
Use the clues. Write the name of each ride next to each vertex on
the map.
Clues:
a. The distance from the ferris wheel to the loop-the-loop is 40 meters.
b. The merry-go-round is 30 meters from the entrance.
c. A round trip from the ferris wheel to the roller coaster is 60 meters.
d. The water slide is 50 meters from the roller coaster.
Math Topic/Concept: scale drawings, logic, proportions
Materials: problem hand-out, pencil, paper
Classroom Use: (Introductory/Developmental/Evaluation)
Grade: 7-8
Grade Cluster: (EarlyElem/LateElem/MS-Jr.High/EarlyHS/LateHS)
Illinois Goal: 6, Number and 7, Measurement
Standard: 6C3a, 7C3a
Applied? (1-4): 3
Source: Greenes, Findell. Groundworks: Algebra
Puzzles and Problems, Grade 7. 1998 Creative Publications. ISBN: 0-7622-0560-1
Answer:
Strategies Listed: Use proportions, use logic to follow
the clues.
Solution: Use proportions to convert each map distance in centimeters
to actual distance in meters. Using clues:
· the merry-go-round is one of the 2 points that are 4.5 cm
from the entrance.
· Only the ferris wheel and roller coaster are 4.5 cm apart.
· The ferris wheel and loop-the-loop must be 6 cm apart. This
locates the ferris wheel and the loop-the-loop. With that information,
locate the roller coaster and merry-go-round.
· The water slide is 7.5 cm from the roller coaster.
Intended rubric or assessment method: ISAT “student friendly”
rubric
Write-up submitted by: M.K. Robbins
Title: Cube Sculptures
Problem: You have studied cubism in art appreciation class.
Your assignment now is to create sculptures made with 16 cubes. The
visible faces of each cube will be painted a different color. How
many different colors of paint will you need?
· Work with a partner. Use linker cubes to design models
of several different sculptures, each containing 16 cubes.
· Determine the number of colors needed to paint each sculpture:
remember, each face must be a different color.
· Draw your sculptures on isometric dot paper. Record
the volume and surface area of each sculpture, using the edge of one block
as one unit.
· Try to make one model for at least one sculpture for every
possible number of visible faces. Be ready to discuss your findings.
Math Topic/Concept: surface area, and volume
Materials: linker cubes, about 70 per pair of students
Isometric dot paper
Classroom Use: (Introductory)
Classroom use comments*: This might be used to introduce
concepts of surface area and volume. Assessment in that case would
be informal, with a journal writing entry for individualized assessment.
Grade: 7 - 8
Grade Cluster: (MS-Jr.High)
Illinois Goal: 7: measurement
Standard: 7C3b
Applied? (1-4): 2
Source: Super Source: 7-8, Measurement; Cuisenaire, 1999.
ISBN: 1-57452-172-1
Answer: There are 14 possible surface areas using 16 cubes
ranging from 40 to 66 square units.
Strategies Listed: building models, drawing models
Intended rubric or assessment method: Journal write: Suppose
you were building sculptures made from 20 cubes. Describe how you
would build the models with the greatest and the least surface areas.
Explain how you would know that these were the greatest and the least.
Write-up submitted by: M.K. Robbins
Title: Building a Snow Statue
Problem: When the snow stopped falling, Kelly, Miguel, and Neha
rushed outside to build a snow statue. Kelly made one large snowball for
the head. Miguel made a larger snowball for the middle section. Neha made
a giant snowball for the bottom section.
As they were getting ready to put the snow statue together,
the children noticed that the circumference of the smallest section was
about 2/3 the circumference of the middle section. The middle section was
about 3/4 the circumference of the largest section. When they measured
the smallest snowball, they found that it had a circumference of about
80 centimeters.
How tall will the snow statue be when the
sections are piled one on top of the other? Assume that the snow
does not compact.
Bonus: The group would like to
make another snow statue with a total height of at least two meters. If
all other conditions are kept the same, what circumference will they need
for the smallest section?
Note: Please be sure to use the
appropriate units of measurement, and state your final answer in a complete
sentence.
Math Topic/Concept: measurement: circle circumference/ diameter
Materials: calculator, pencil, paper
Classroom Use: (Developmental)
Classroom use comments*: Students may need help with appropriate
rounding, and making sense of answers rather than depending on calculator
output of long decimal numbers. Using 3.14 or 22/7 for pi work equally
well. The pi key on a calculator may give less user-friendly results.
Grade: 7 - 8
Grade Cluster: (MS-Jr.High)
Illinois Goal: 7: Measurement & 9: Geometry
Standard: 7C3b & 9C3b
Applied? (1-4): 3
Source: www.forum.swarthmore/libr
Swarthmore University’s past problems of the week
Answer: The snow statue will be about 115 cm tall.
Bonus: the smallest snowball will need a circumference that is about 140
cm.
Strategies Listed: drawing, algebraic formulas
Solution: Small snowball has C = 80 cm. Middle snowball
C = 120 cm, since the small is 2/3 of the middle. The middle is 3/4
the size of the large, so the large snowball's C = 160 cm. The diameter
of each = C / pi. Using pi = 3.14, you get diameters of about 25.478, 38.217,
and 50.956 cm. Stacked as a snowman, the height is about 115 cm.
Extensions or related problems*: How many rotations will
your 26-inch bicycle tire make in a fifteen mile bicycle trip?
Intended rubric or assessment method: ISAT “student friendly”
rubric
Write-up submitted by: M.K. Robbins
Title: Wrapping Paper
Problem: You work for Gourmet Chocolate Company.
Each piece of candy is packaged in a single cube shaped box. Two
dozen boxes are packaged in each rectangular shaped carton. How many
different carton designs are possible?
· Work with a partner. Each linker cube represents a box with
one piece of candy. Build three different cartons, each containing
two dozen candy boxes. The cartons must be rectangular.
· Find the volume and surface area of each carton. The
length of the edge of one cube is 1 unit.
· Draw each of your cartons and record its volume and surface
area on isometric dot paper.
· Use snap-cube grid paper to draw a one-piece pattern that
can be folded to wrap each carton. Use dotted lines for fold lines,
and do not allow for any overlaps.
· Cut out your patterns and fold them to check that they wrap
around your cartons properly. Make needed changes to your patterns
so that they work.
· Record the total number of square units of wrapping paper
needed to make each of your patterns.
· Note any patterns in your data and be ready to discuss your
findings.
Math Topic/Concept: surface area, volume, nets
Materials: linker cubes, isometric dot paper, snap cube
grid paper, scissors
Classroom Use: (Developmental)
Grade: 7-8
Grade Cluster: (MS-Jr.High)
Illinois Goal: 7: measurement
Standard: 7C3b
Applied? (1-4): 3
Source: The Super Source: 7-8, Measurement; Cuisenaire,
1999. ISBN: 1-57452-172-1
Answer: There are 6 different rectangular cartons with a
volume of 24 cubic units. Surface areas range from 52 to 98 square
units.
Strategies Listed: physical models, drawings
Solution: Record data from all groups in the class, 1 design
per group until all are listed.
Extensions or related problems*: Do the same activity,
but do not require cartons to be rectangular prisms. Include nets
to wrap odd-shaped solid stacks of cubes.
Intended rubric or assessment method: Informal class discussion:
· How did you determine surface area and volume of cartons?
· How do we know whether we have found all possible carton shapes?
· How did you go about designing the wraps for your cartons?
· What patterns did you notice in our class data?
· How are the measurements you found related to the dimensions
of each box?
Notes*: Journal entry: Suppose you know the length, width,
and height of a rectangular solid, but do not have a model. Explain
how you would design a net for the solid, and how you would determine how
much paper would be needed to make the net.
Write-up submitted by: M.K. Robbins
Back to
Problem-Solving Database Chart
James R. Olsen, Western Illinois University
E-mail: jr-olsen@wiu.edu
updated June 27, 2001
